Tag: Democrat

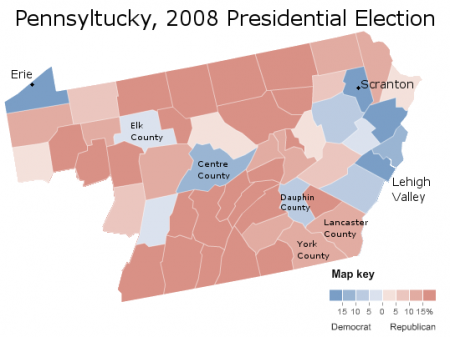
Illinois is losing one congressional district this year – going from 19 to 18.  I have created a map that takes the current map with a delegation of 11 Republicans and 8 Democrats to one with 12 Democratic seats and 6 Republican ones.  I consider this an ambitious gerrymander favoring the Democrats, but one that is realistic and keeps a few things in mind:

1.) Michael Madigan is in charge and he will look out for his interests first.  He also will never do a dummymander.

2.) The Democratic incumbents also have input and their interests will be considered.  None of them will want their district lines to change much and all want their Democratic primary bases to be kept intact.  They also don’t want to be thrown from safe seats to possibly competitive ones.

3.) African Americans will insist on maintaining their three black majority districts, no matter how much population they have lost in the city.  The Hispanics will want at least one – two may be forced by the courts – but Madigan and his allies will likely push for one Hispanic district to maintain Lipinski’s seat.  Also, we have seen in the City of Chicago that Hispanic majority seats often go to white incumbents who control the Democratic machine.  Alderman Ed Burke’s 14th ward is 88% Hispanic, Madigan’s 13th ward is 72% Hispanic and just elected somebody named “Marty Quinn” to be Alderman.  

4.) Madigan will go after Freshman GOP before those with more seniority.  Not only are they easier targets, but having seniority on both sides of the aisle is good for Illinois.

Below is each new district with data and analysis.  I have calculated the results from the 2010 Senate race (Kirk v. Giannoulias) and from the 2004 Presidential race (Bush v. Kerry – I know, old).  I used the 2010 Senate race rather than the Governor’s race because it is on the Federal level, and to use data against moderate Republican.  I did not calculate data from the 2008 election, because Obama’s landslide was far too big  and unevenly distributed in Illinois (I feel Chicagoland was much more skewed than Downstate).  The 2010 numbers are exact (to the precinct) except for Tazewell, Marion, Moultrie, and Menard Counties, which I allocated votes by ratio of population in each district.  The same goes for 2004 numbers, except I had to extrapolate the precinct data for Lake and Will counties from 2010 data.

For now, here are three tables with election data, racial population data, and VAP data.  I will add more analysis in the next few days.

District  Kirk   Alexi    Bush   Kerry    PVI

1        24.80% 75.20% 23.00% 77.00% D+30

2        25.26% 74.74% 26.39% 73.61% D+28

3        48.14% 51.86% 44.25% 55.75% D+8

4        26.57% 73.43% 26.90% 73.10% D+28

5        47.72% 52.28% 41.53% 58.47% D+10

6        63.33% 36.67% 57.66% 42.34% R+6

7        18.61% 81.39% 15.81% 84.19% D+37

8        53.71% 46.29% 46.66% 53.34% D+4

9        47.77% 52.23% 39.12% 60.88% D+11

10       47.75% 52.25% 39.70% 60.30% D+11

11       68.75% 31.25% 60.55% 39.45% R+10

12       53.24% 46.76% 47.02% 52.98% D+4

13       49.12% 50.88% 45.12% 54.88% D+7

14       64.94% 35.06% 59.55% 40.45% R+8

15       68.33% 31.67% 59.68% 40.32% R+10

16       67.03% 32.97% 57.86% 42.14% R+8

17       56.61% 43.39% 46.84% 53.16% D+3

18       68.31% 31.69% 60.39% 39.61% R+10

ALL

District  White  Black  Hispanic  Asian

1        35.5%   52.7%   8.2%   2.0%

2        31.4%   52.8%   13.4%   0.8%

3        59.5%   6.1%   27.7%   5.4%

4        18.7%   4.1%   73.3%   2.9%

5        65.1%   2.2%   24.4%   6.7%

6        75.4%   3.7%   8.8%   10.3%

7        27.6%   54.5%   12.2%   4.1%

8        48.6%   8.7%   31.5%   9.1%

9        67.4%   7.8%   10.6%   12.0%

10       72.2%   3.4%   10.9%   11.5%

11       92.7%   3.2%   2.0%   0.8%

12       77.5%   16.6%   2.8%   1.1%

13       53.0%   12.7%   27.3%   5.0%

14       82.5%   1.6%   11.4%   3.2%

15       85.4%   6.8%   3.1%   2.9%

16       88.6%   2.6%   6.3%   1.1%

17       77.9%   12.4%   5.8%   1.5%

18       86.7%   5.4%   4.8%   1.6%

VAP

District  White  Black  Hispanic  Asian

1        38.0%   51.7%   6.9%   2.2%

2        34.5%   51.8%   11.7%   0.8%

3        64.0%   5.9%   23.5%   5.7%

4        23.2%   4.1%   68.3%   3.4%

5        69.1%   2.0%   20.9%   6.8%

6        77.7%   3.5%   7.5%   10.2%

7        31.1%   51.8%   11.0%   4.8%

8        54.1%   8.1%   26.9%   9.4%

9        69.2%   7.8%   9.3%   12.1%

10       74.7%   3.3%   9.3%   11.2%

11       93.5%   3.2%   1.7%   0.8%

12       80.0%   15.3%   2.4%   1.1%

13       58.3%   12.1%   23.4%   5.0%

14       85.3%   1.4%   9.4%   3.1%

15       86.7%   6.2%   2.7%   3.2%

16       90.8%   2.4%   4.9%   1.1%

17       81.7%   10.8%   4.6%   1.5%

18       88.7%   5.1%   3.8%   1.5%

A hyper-partisan Democratic gerrymander of Texas.  Probably not VRA compliant and Texas might even have some laws against such whacked-outness.  However when drawing hyper-partisan maps I like to take “screw the other party over as much as possible and forget the law” approach.  This another full Texas map, the first I drew was before partisan data was available.

The two crowning achievements of this map are the three Democrats out of Travis County (City of Austin), which is something I posted earlier and worked to further perfect, and six Democrats out of Harris County (City of Houston).  

Every Republican seat is a major vote sink, only 4 of the 14 GOP seats are less than 70% McCain.  Which means there are 10 seats that are R+24 or higher including what would be the most Republican district in the country at R+30.

For the sake of classifying districts 59% Obama and higher are labeled as Safe D, 56-58% is Likely D and <56% is Lean D.  There is only one district that is less than 56% Obama than isn’t a GOP vote sink.

Overall this is a 22-14 Democratic Map with one district that could be won by the GOP in a wave year.  So I think the worst case scenario for the Dems would be 21-15

District 13

Obama 23%

McCain 76%

69% White, 6% Black, 22% Hispanic

The most Republican district in the state and probably the most conservative in the country.  Interestingly enough despite being a R+30 district it is 22% Hispanic.

Safe R

District 17

Obama 26%

McCain 73%

67% White, 5% Black, 26% Hispanic

A R+27 West Texas District that is 26% Hispanic.  Includes the cities of Midland, Odessa and San Angelo.

Safe R

District 19

Obama 27%

McCain 72%

67% White, 6% Black, 25% Hispanic

This is a third West Texas district that is over 20% Hispanic.  That definitely doesn’t help in this district.  Includes the cities of Abilene and Lubbock

Safe R

 

District 4

Obama 31%

McCain 68%

81% White, 8% Black, 8% Hispanic

North East Texas and part of Collin County

Safe R

District 5

Obama 28%

McCain 71%

76% White, 13% Black, 9% Hispanic

Tyler-Longview based district for Louie Gohmert.  Hey we got to keep the most entertaining Republicans right!

Safe R

District 8

Obama 26%

McCain 73%

81% White, 4% Black, 11% Hispanic

Includes most of the very fast growing Montgomery County.

Safe R

District 11

Obama 28%

McCain 71%

74% White, 8% Black, 14% Hispanic

This is my favorite GOP vote sink based on unattractiveness.  It gets all the Republican vote that would have ended up jeporadizing district 10, 31 and 9 if no counties were split.

Safe R

District 14

Obama 29%

McCain 70%

73% White, 6% Black, 17% Hispanic

Another GOP vote sink near Houston.  

Safe R

District 25

Obama 27%

McCain 72%

78% White, 13% Black, 8% Hispanic

East Texas GOP district.  

District 35

Obama 26%

McCain 73%

78% White, 6% Black, 11% Hispanic

Like the rest of the GOP districts, another vote sink carefully drawn.

District 16

Obama 59%

McCain 40%

24% White, 3% Black, 70% Hispanic

Most of El Paso but I also used 16 to grab some of the more Republican counties from district 23 in order to help make 23 more Democratic.

Safe D

District 23

Obama 55%

McCain 44%

27% White, 2% Black, 69% Hispanic

Shedding some of the more Republican counties and picking up part of Southern El Paso shifts this district 4 points towards the Democrats compared to it’s previous 51% Obama/48% McCain breakdown.  In 2010 Conseco beat Rodriguiz by 5 points.

Lean D

District 36

Obama 29%

McCain 70%

80% White, 2% Black, 16 Hispanic

Contains a decent amount of Lamar Smiths current district including the conservative parts of North Bexar County.

Safe R  

District 15

59% Obama

40% McCain

19% White, 1% Black, 78% Hispanic

Most of McAllen and the most Republican parts of Corpus Christi are here.  

Safe D

District 27

Obama 58%

McCain 41%

22% White, 2% Black, 74% Hispanic

Solomon Ortiz’s loss to Blake Farenthold was definitely a sign of a GOP wave.  The old district 27 was 53%/46%.  I would want to rate this district Safe D since Farenthold won by 1 point and the shift by 5 probably would have safed Ortiz, but I’d be breaking break my own rules.

Likely D

District 28

Obama 59%

McCain 41%

19% White, 3% Black, 76% Hispanic

This district shifts a few more points Democratic from his current 56%/43% district.  It was difficult to get a good image capture of this district since it is drawn like it is.

Safe D

District 20

Obama 58%

McCain 41%

30% White, 5% Black, 61% Hispanic

I tried so hard to get two 59% Obama districts in San Antonio and just couldn’t do it.  58% is really close and as much as I want to label this a safe D district I can’t since my intro says 59% will be considered a safe D district.

Likely D

District 21

Obama 58%

McCain 41%

33% White, 10% Black, 54% Hispanic

Eastern San Antonio and southern Bexar county.  

Likely D

I would have zoomed in more but that would have cut off several of the districts originating from Houston.

District 2

Obama 59%

McCain 40%

36% White, 26% Black, 33% Hispanic

Very Democratic part of North Houston and the Democratic parts of Huntsville in East Texas

Safe D

District 7

Obama 59%

McCain 40%

35% White, 24% Black, 30% Hispanic, 11% Asian

District 9

Obama 59%

McCain 41%

38% White, 27% Black, 23% Hispanic, 12% Asian

Stretches from Galveston up into Brazos County, most of the Democratic vote is in Fort Bend.  Interesting note is this district is 12% Asian

Safe D

District 18

Obama 59%

McCain 40%

39% White, 25% Black, 28% Hispanic

South and West Houston district.

Safe D

District 22

Obama 60%

McCain 40%

39% White, 32% Black, 26% Hispanic

The most Democratic district partially in Houston also includes Beaumont, Port Arthur, Hudson and Nacodoches.  

Safe D

District 29

Obama 59%

McCain 40%

29% White, 10% Black, 57% Hispanic

A good piece of Green’s current district is in the new 29, he should have no problem winning here.

Safe D

Like Houston I had to take this photo to account for the sprawly districts

District 10

Obama 60%

McCain 39%

52% White, 6% Black, 38% Hispanic

This district includes most of Lloyd Doggetts current district in South Austin and it sort of stretches all the way down into South Texas.  

Safe D

District 31

Obama 58%

McCain 40%

47% White, 24% Black, 25% Hispanic

Squeezing a third Democratic district out of Travis County was great.  This district includes parts of Travis County, Waco, Killeen, Temple, College Station

Likely D

District 33

Obama 59%

McCain 40%

67% White, 7% Black, 19% Hispanic

Most of Austin is in this district including Round Rock.

Safe D

District 3

Obama 60%

McCain 38%

39% White, 18% Black, 38% Hispanic

The most Democratic district in the state with Obama winning by a 22 point margin.  It loops around the very conservative Park Cities and Preston Hollow area of Dallas County

District 12

Obama 59%

McCain 40%

45% White, 21% Black, 29% Hispanic

Contains much of the City of Fort Worth.  I’d like to see Lon Burnam run in this one.  He’s a very liberal State Representative in Fort Worth.

Safe D

District 24

Obama 59%

McCain 40%

53% White, 18% Black, 21% Hispanic

Contains the Democratic parts of Arlington in Tarrant County and Southwest Dallas County.   The college town Denton is also roped into the district.

Safe D

District 30

Obama 60%

McCain 39%

40% White, 19% Black, 35% Hispanic

Mostly West Dallas including southern parts of the city and Democratic pieces of Collin/Denton County

Safe D

District 32

Obama 60%

McCain 39%

45% White, 28% Black, 21% Hispanic

South Dallas and some eastern Dallas suburbs including Garland.  I think Eddie-Bernice Johnson would run in this one.

Safe D

Well it happen?  No, but a Democrat can dream 🙂

Everyone who gerrymanders knows about the infamous GOP cracking of Austin during the GOP gerrymander of Texas.  If the Democrats magically got control of the state House, state Senate and Governorship they would be out for blood over the GOP mid-decade gerrymander

Time for the GOP’s turn to cry over a gerrymander of Austin!

CD-10

Obama 59%

McCain 40%

White 55% Black 6% Hispanic 35%

Since Doggett’s District has grown by over 120,000 people in the past 10 years it has been easy to make it more compact by removing several of the conservative counties, some of Austin was also removed to make room for the other districts.  Austin comprises the majority of the population, but San Marcos and Seguin are also in the district.  The PVI remains the same as Doggett’s old district.  If he can win with an 8 point margin in one of the worst years for Democrats in decades this new district should be no problem.  

Safe Democrat

CD-33

Obama 60%

McCain 39%

White 65% Black 8% Hispanic 20%

Clearly most of Austin is in this new district.  It is 2 points more Democratic than Doggett’s district and includes Round Rock and Georgetown in addition to the majority of Austin.  Again the massive population growth in Austin made this district possible.  Not much else to say on this one.

Safe Democrat

CD-31

Obama 56%

McCain 43%

White 52% Black 21% Hispanic 21%

As if two safe Democratic districts weren’t bad enough a third district including parts of Austin, Waco, Killeen/Temple and College Station/Bryan.  This is the crown jewel of this gerrymander.  This district is beautifully drawn for one reason, to get the GOP angry. I couldn’t quite make this district Democratic enough to be a safe Democratic Seat, but it is definitely winnable, toss-up at worst and lean D at best.

Lean D/Toss-Up

Had a whole write up posted but it got deleted. No comments this time but you guys know the deal by now. Its a 17-16-3 Democratic gerrymander.

http://img.photobucket.com/alb…

http://img.photobucket.com/alb…

El Paso: http://img.photobucket.com/alb…

District 1 (Blue) 54 Obama 66 Hispanic

El Paso and Odessa

Lean/Likely D

District 2 (Green) 57 O 72 H

El Paso and tentacles

Likely D

District 3 (Purple) 56 O 78 H

Mexican Border and some other counties

Likely D

District 4 (Red) 55 0 74 H

Mexican Border and it snakes up

Lean/Likely D

District 5 (Yellow) 55 0 72 H

Mexican Border and Corpus Christi

Likely D

District 6 (Teal) 34 O 59 White

Counties that border the Gulf of Mexico and going inward.

Safe R

San Antonio: http://img.photobucket.com/alb…

District 7 (Gray) 55 O 58 H

Southern San Antonio and surrounding areas

Likely D

District 8 (Lilac Purple) 54 O 54 H

Middle of San Antonio and some other parts of Bexar county.

Lean/Likely D

District 9 (Sky Blue) 33 O 69 W

Northern San Antonio and its conservative suburbs and exurbs.

Safe R

Austin: http://img.photobucket.com/alb…

District 10 (Bright Pink) 55 O 57 W

Austin and its suburbs

Likely D

District 11 (Lime Green) 54 O 63 W

Austin

Lean/Likely D

District 12 (Periwinkle) 53 O 67 W

Austin and Killeen

Lean D

District 13 (Burt Sienna) 31 O 74 W

Waco, Temple and empty spaces

Safe R

District 14 (Gold) 25 O 66 W

A lot of space and part of lubbock

Safe R

District 15 (Orange) 25 O 66 W

More Nothing.

Safe R

Fort Worth: http://img.photobucket.com/alb…

District 16 (Kelly Green) 52 O 51 W

Surprisingly Conservative Fort Worth

Toss-Up

District 17 (Navy Blue) 30 O 84 W

Suburbs of Fort Worth

Safe R

Dallas: http://img.photobucket.com/alb…

District 18 (Golden Yellow) 59 O, 30 Black 19 Hispanic 5 Asian

Dallas

Safe D

District 19 (Puke Green) 54 O, 22 Black 27 Hispanic 2 Asian

Dallas and a few southward counties

Likely D

District 20 (Rose) 55 O, 39 Hispanic 14 Black 6 Asian

Dallas and Irving

Likely D

District 21 (Blood Red) 50 O, 54 W

Dallas  and surrounding areas

Toss-Up

District 22 (Poo Brown) 39 O 72 W

North of Dallas and FW

Safe R (Winnable with a great candidate in a good year or an incumbent in an ok one, but tilts very r)

District 23 (Robin’s Egg Blue) 35 O 75 W

North of FW to OK border

Safe R

District 24 (Dark Purple) 29 O 72 W

Wraps Around 19, 13 to the end of 11

Safe R

District 25 (Salmon)  35 O, 79 W

North Of Dallas

Safe R

District 26 (Dark Gray) 33 O, 77 W

Between OK Border and 24

Safe R

District 27 (Seafoam Green)31 O 72 W

Along the LA Border

Safe R

Houston: http://img.photobucket.com/alb…

Note: I made a small error here in the large map. It is fixed in the numbers and in the close–up of houston (part of the district didn’t connect)

District 28 (Really Light Purple) 58 O  28 Black 13 Asian 26 Hispanic

Southwest Houston

Safe D

District 29 (Sage Green) 33 O 73 W

Northeast of Houston

Safe R

District 30 (Pinkish-Red near houston) 33 O 70 W

Wraps Around Houston

Safe R

District 31 (Pale Yellow) 39 O, 61 W

South of Houston

Safe R (See District 22)

District 32 (Bright Red) 37 O, 66 W

Wraps even closer around Houston then does 30

Safe R

District 33 (Denim Blue) 56 O, 24 B 7 A 28 H

Houston

Likely D

District 34 (Green in Houston) 51 O, 12 B 6 A 34 H

Houston

Toss-up

District 35 (Purple in Houston) 53 O, 16 B 3 A  43 H

North Houston

Lean D

District 36 (Orange in Houston) 62 O, 26 B 42 H

Southeast Houston riding up to Northwest Houston

Safe D

What I think makes this most effective is that the Democratic districts will only get more liberal over the Decade, cementing the safety of Incumbents and helping unseat any republicans who manage to pull an upset or win a toss-up.

Please reply, this took a while 🙂

And a big thank you for my girlfriend for help with the names of colors (You can tell which parts she left for, lol)

Bill White, the former Mayor of Houston, is sitting on $5.4 million for the general election.  Roughly twice as much as either Rick Perry or Kay Bailey Hutchinson.  

Kay Bailey Hutchinson

1.1M raised

8M spent

2.3M Cash on Hand

Rick Perry

850K raised

8.8M spent

2.5M CoH

Debra Medina

450K raised

228k spent

291K CoH

Bill White

2.2M raised

2.7M spent

5.4M CoH

Farouk Shami

1.1M raised

5M spent

1M CoH

Thanks to the BurntOrangeReport for the numbers

http://www.burntorangereport.c…

This is the fifth part of an analysis of the swing state Pennsylvania. It focuses on the traditionally Republican region between the Democratic strongholds in the southeast and southwest. The last part can be found here.

Pennsyltucky

Outside the Pittsburgh and the Philadelphia metropolis, Pennsylvania is a very different place. Political analysts often label this area “the T,” while others call it Pennsyltucky.

Popular culture mythologizes Pennsyltucky as red-neck capital – a rural region dominated by NASCAR-loving red-necks. Politically, James Carville compared Pennsyltucky to Alabama without the blacks.

In fact, this stereotype is inaccurate on two accounts. Firstly, Pennsyltucky contains far more than so-called rural red-necks; most of its counties are fairly populated (they are far more densely peopled than, say, rural Arkansas). Secondly, many of these supposedly NASCAR-loving red-necks also belong to the local union and vote Democratic on economic issues. The majority may support Republicans, but that majority certainly is below the 88% of Alabama whites that voted for John McCain.

Nevertheless, the “T” does constitute the Republican base in Pennsylvania. Former president George W. Bush pulled 48.42% of the state’s vote in 2004, and he had to get those votes somewhere.

More below.

Pennsylvania’s 2006 Senate election provides a geographic illustration of this base. In that election, former Senator Rick Santorum lost by a landslide 17.36% margin; only the reddest counties supported him:

Although they cover a lot of land, not all these counties are rural enclaves of Pennsyltucky (if they were all rural, Senator John Kerry would have won by double-digits in the state). In fact, fast-growing exurbs constitute a substantial source of Republican votes. Located east of the Philadelphia metropolis, these are somewhat wealthy and mostly white. They include Lancaster County (where Bush won 65.80% of the vote) and York County (where he won 63.74%); the former president came out of these two counties with a 121,832 margin, enough to offset Pittsburgh, Erie, and Scranton.

Erie and Scranton both constitute solidly blue areas belonging to “the T.” They give lie to the myth that all Pennsyltucky votes loyally Republican. Like the southwest, Erie and Scranton contain a number of working-class Democrats; unlike the southwest, however, cultural appeals have not swayed these folk into voting Republican.

Indeed, Democrats do respectably in many parts of Pennsyltucky. Here is President Barack Obama’s performance:

Mr. Obama did not just win Erie and Scranton; he took several other counties and ran closely elsewhere. These included Centre County, home to Pennsylvania State University, and Dauphin County, which has a relatively high black population. All the Lehigh Valley – somewhat an extension of Philadelphia’s suburbs – voted for the president. More surprisingly, Obama ran very closely in several rural, lily-white regions of the T; one such county (Elk) even gave the president a 4% margin of victory.

Obama was not the only Democrat to do well in parts of Pennsyltucky. Here is how former president Bill Clinton performed:

Mr. Clinton, of course, was a fellow with immense appeal to so-called “red-necks.” Since his time, much of Pennsyltucky has moved to the right. Yet not all of it is deep-red: while some counties gave Mr. McCain more than 70% of the vote, others – demographically identical – gave him barely more than 50%. These are substantial and curious variations.

While Pennsyltucky as a whole votes strongly Republican, it is wrong to generalize the area. Its most populous regions – the exurbs – constitute a vital part of the Republican coalition, while some rural counties have a fairly weak Republican habit. Finally, a number of places dependent upon industry routinely support Democrats. To stereotype the “T” as a composed solely of Republican-voting red-necks would do injustice to the region’s complexities.

(Note: All statistics come from http://uselectionatlas.org/ . Some pictures modified from the NYT.)

–Inoljt, http://mypolitikal.com/

This is the fourth part of an analysis of the swing state Pennsylvania. It focuses on the industrial southwest, a once deep-blue region rapidly trending Republican. Part five can be found here.



Pittsburgh and the Southwest

Pennsylvania’s southwest has much in common with West Virginia and Southeast Ohio, the northern end of Appalachia. Electoral change in the region is best understood by grouping these three areas together as a whole.

Socially conservative (the region is famously supportive of the NRA) but economically liberal, the industrial southwest voters typify white working-class Democrats. These voters can be found in unexpected places: Catholics in Rhode Island and Massachusetts, loggers along the Washington coast, rust-belt workers in Duluth, Minnesota and Buffalo, New York.

It was President Franklin D. Roosevelt and the New Deal that brought the working-class to the Democratic Party; before his time, the party constituted a regional force confined mainly to the South. In Pennsylvania, a Republican stronghold that had voted for President Herbert Hoover, Mr. Roosevelt laid the foundations for a lasting Democratic coalition.

For decades, voters in southwest Pennsylvania constituted this coalition’s foundation. Take, for instance, Democratic nominee Walter Mondale:

In 1984, the industrial southwest, badly hurting from a receding recession, cast a strong ballot for Mr. Mondale. It did so again for Governor Mike Dukakis, and twice for President Bill Clinton.

Ironically, it was during the presidency of Mr. Clinton – a man much liked by Appalachia – that the Democrats became regarded as the party of the coasts and the elite. Ever since his time, Pennsylvania’s industrial southwest has been in a bad way for Democrats.

More below.

Thus, whilst metropolitan Philadelphia has been moving steadily left, Pittsburgh and the industrial southwest have been marching in the opposite direction.

To get a sense of the movement in this region, compare these two maps:

In less than a generation’s span, one sees Democratic strength in northern Appalachia utterly vanish.

In a state where things have been going badly for Republicans, southwest Pennsylvania provides some consolation. Were it not for the southwest’s rightward trend, Pennsylvania would today be a fairly solid Democratic state.

Nevertheless, if I were to choose between Pittsburgh and the industrial southwest or Philadelphia and the suburban southeast, I would much prefer the latter. While Philadelphia itself is in declining, its metropolitan area as a whole has experienced rapid growth. The southwest’s population, on the other hand, remains basically stagnant, suffering the effects of economic decline.

In absolute terms, moreover, eastern Pennsylvania holds far more votes:

Republicans might take comfort in Allegheny County’s vote reservoir – were it not consistently blue. Indeed, Democratic strength in Pittsburgh ensures that, as a whole, the southwest will still vote Democratic for some time yet. Although – unique to practically every other major city – Republicans have been improving in Pittsburgh, its substantial black population limits their potential.

The puzzling thing, however, is why Appalachian working-class whites are moving so rapidly right. It cannot be simply race: both Vice President Al Gore and Senator John Kerry were white, after all, yet they still did progressively worse. It cannot be simply elitism, either: Governor Mike Dukakis and Governor Adlai Stevenson were intellectual technocrats, yet they won what Mr. Kerry and Mr. Gore could not.

Finally, it is not as if all the white working-class has suddenly turned Republican: voters in Michigan, northeast Ohio, upstate New York, and Silver Bow and Deer Lodge Montana, amongst other regions, still retain the Democratic habit. In Pennsylvania, working-class strongholds such as Scranton and Erie, surrounded by a sea of Republican counties, also continue to vote deep blue. They will be the topics of the next post.

–Inoljt, http://mypolitikal.com/

Dan Seals announced his candidacy to seek the democratic nomination for IL-10 this morning.  

With Kirk running for Senate, it’s not too surprising that Seals decided to run again.  But whether his previous elections will give him a leg up in the race will be an interesting development to find out

Here is an article from Lynn Sweet of the Chicago Sun-Times:

http://blogs.suntimes.com/swee…

Crossposted from Michigan Liberal

Friends,

First, I’d like to begin by thanking all of you for your support. Because of contributions from people like you, our campaign has just been ranked in CQ’s list of the top ten best funded challengers in the country. The voters in Michigan’s 9th Congressional district are tired of the failed leadership they’ve gotten from my opponent, Congressman Joe Knollenberg, for the past sixteen years, and they’re eager for a real change in Washington.

As a former Lt. Commander in the Navy, I wanted to take a moment to write to you this week as we pass the fifth anniversary of one of the most shameful moments in recent American history. On May 1st, 2003, President George W. Bush landed on the USS Abraham Lincoln, in front of the now-infamous “Mission Accomplished” banner and said these words: “My fellow Americans, major combat operations in Iraq have ended. In the battle of Iraq, the United States and our allies have prevailed.”

More after the break. 

Five years later, another 3,919 brave American soldiers have been killed in combat in Iraq, the country has descended to the brink of all out civil war, and the Republican candidate for President has suggested the possibility of remaining in Iraq for another hundred years.

Five years later, we have more troops in Iraq today (155,000) than we did back then (150,000).

Five years later, we are spending $1 billion every two and a half days in Iraq – money that we could be investing in jobs, infrastructure, and rebuilding our economy here at home.

Five years later, even though it is tragically clear that the mission was not accomplished, Congressman Knollenberg has continued to vote for President Bush’s failed policies. Our men and women in uniform deserve real leadership and a responsible plan to start bringing them home now.

We have also not accomplished the mission of taking care of our troops and veterans when they return home from combat. The brave men and women who have sacrificed for our country deserve the best health care we can provide – instead we have given them the Walter Reed debacle and a VA system with a backlog of more than half a million benefits claims.

This failure of leadership for our veterans is having terrible consequences. Last week, the VA confirmed a truly appalling statistic – an average of eighteen veterans commit suicide every day. At that rate, over 6,500 brave men and women who served and sacrificed for our country will take their own lives this year. That’s nearly 1,500 more than the total number of soldiers who have been killed in combat in Iraq.

Sadly, the reasons for this situation are all too clear. A recent study by the American Psychological Association reported that over 32% military personnel who had been deployed to war zones said that they suffered a ‘negative impact’ on their psychological well-being – but only 1 in 10 sought treatment for mental health concerns. The rest stayed quiet, out of embarrassment, or fear that, if they asked for help, their military careers would be in jeopardy.

As a veteran, I take this very personally. Quite simply, the failure of our leaders in Washington to care for our veterans may be the greatest, unspoken tragedy of the entire Iraq war debacle. The failure to reach out to those veterans who may not have physical injuries – but whose psychological wounds can be even more severe – is not only a betrayal of the sacred trust between our nation and our veterans, but it has serious repercussions on our long-term military readiness and security.

Care for our veterans is a fundamental, guiding principle of our democracy that our greatest leaders have always understood. In his second inaugural address, Abraham Lincoln spoke of our duty to “…care for him who shall have borne the battle and for his widow and his orphan….” Three quarters of a century earlier, in 1789, George Washington put it this way: “The willingness with which our young people are likely to serve in any war, no matter how justified, is directly proportional to how they perceive the veterans of earlier wars were treated and appreciated.”

When Democrats took control of Congress in 2006, they started to address the serious failures in our VA and military health care system. Despite the objections of the President, Democrats passed the largest funding increase in the history of the VA. This was a good start, but we still need to do much more to care for our soldiers, veterans, and their families – and that will take electing real leaders to Congress, and replacing Bush Administration rubber stamps like Congressman Knollenberg.

When the House voted on a bill to guarantee that soldiers would have at least as much time at home as they spend deployed overseas, Congressman Knollenberg voted no.

When the House took up a bill that included $3.3 billion to improve military medical care, $1.8 billion for veterans care, and that would have started withdrawing troops this summer, Congressman Knollenberg voted no.  After that bill was vetoed by the President, Congressman Knollenberg voted to sustain the veto.

In Washington, I will work to bring about a responsible end to the war in Iraq and start bringing our troops home now. As a veteran, I will be a voice for a strong foreign policy that keeps America secure and takes the fight to our real enemies, and I will do everything in my power to ensure that we keep faith with the men and women who served and sacrificed for our great nation.

Real leadership means taking action to solve our problems – not passing the buck and waiting for someone else to act – and that’s what I will deliver in Washington. Five years after ‘Mission Accomplished,’ it’s long overdue.

I’m not going to do this alone. This campaign to unseat one of the biggest obstacles to change in Congress will take a group effort. We’re doing well now, but Congressman Knollenberg is going to be very well funded by entrenched special interests who don’t want to lose their inside man in the House Appropriations Committee. If we’re going to be able to fight back against the GOP smear machine and the negative attacks ads that we all know are coming, and if we’re going to bring real leadership to Michigan’s 9th District, I will need your help. 

Please visit http://www.petersforcongress.com/ to learn more, or click here to join the effort by contributing on ActBlue.

The last 4 months have been exciting and rewarding to travel around the 5th district campaigning.  I’ve learned a lot about the district, the people and myself in the process.  It all came to a climax on Tuesday, April 22nd as the voters in the 5th district went to the polls to select who would be the 2 remaining candidates to continue on to the November 4th general election.

From a personal standpoint, I learned that in politics, the best thing you can do is plan your strategy and stay the course.  You have to be honest with the people, explain where you stand on the issues important to the people you want to represent and trust their instincts when they go to the polls.

As Kelly, Amanda and I watched the election returns on Tuesday evening I couldn’t help but wonder if I had done enough to get our message out to the voters in the 5th district.  Kelly was nervous and Amanda’s only concern was “Is daddy winning?”  At the end of the evening, just after 11:00 PM, both of the area TV stations called the election in our favor.  A short time later, both of my opponents called to offer their congratulations and I gave them both thanks for the positive, clean campaigns they both ran.  

Waking up on Wednesday, I have to admit I was still overwhelmed by results from the night before.  The day found many people calling and stopping in the office to offer congratulations.  In the evening, I traveled to Reynoldsville for the monthly meeting of the Jefferson County Democratic Committee.  It was fitting that I was making my first appearance as the Democratic nominee for 5th district at the same meeting I attended as my first event as an official candidate back in January.  

Of course, the reality is that for everything we accomplished on Tuesday, the real work began on Wednesday morning.  It is going to be busy between now and November 4th.  I’ll continue to keep everyone updated on how the campaign is going.  If you know of any events that we should be attending, please email the campaign and let us know.  Also, if you have any ideas to help reach out to the voters please forward them to the campaign.  

I’ll conclude with this.  Most of the media interviews I had on Tuesday night and Wednesday morning all asked “Can you win this seat in November?”  After about the fifth or sixth time I got the question, I finally responded “We can and we will.”

Mark B. McCracken

Your Candidate For Congress

————————————————————————————————–

This diary is cross-posted at McCracken’s campaign blog, PA’s Blue Fifth

Mark McCracken for Congress

ActBlue page